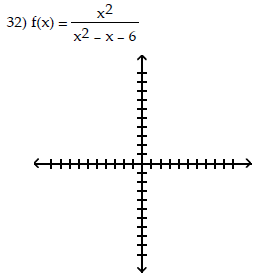Try the Free Math Solver or Scroll down to Tutorials!

Enter expression, e.g. (x^2-y^2)/(x-y)

Enter expression, e.g. x^2+5x+6

Enter expression, e.g. (x+1)^3

Enter a set of expressions, e.g. ab^2,a^2b

Enter a set of expressions, e.g. ab^2,a^2b

Enter equation to solve, e.g. 2x+3=4

Enter equation to graph, e.g. y=3x^2-1

Depdendent Variable

Number of equations to solve:

Equ. #1:

Equ. #2:

Equ. #3:

Equ. #4:

Equ. #5:

Equ. #6:

Equ. #7:

Equ. #8:

Equ. #9:

Solve for:

Enter inequality to solve, e.g. 2x+3>4

Enter inequality to graph, e.g. y<3x^2-1

Dependent Variable

Number of inequalities to solve:

Ineq. #1:

Ineq. #2:

Ineq. #3:

Ineq. #4:

Ineq. #5:

Ineq. #6:

Ineq. #7:

Ineq. #8:

Ineq. #9:

Solve for:

Math solver on your site

Please use this form if you would like
to have this math solver on your website,
free of charge.

Name:

Email:

Your Website:

Msg:

MATH 140 Practice Questions Exam2

Find the domain and range.

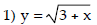
Solve the problem.

2) If a rocket is propelled upward from ground
level, its height in meters after t seconds is
given by h = -9.8t^2 + 127.4t. During what
interval of time will the rocket be higher than
411.6 m?

For the pair of functions, perform the indicated operation.

3) f(x) = 9x + 9, g(x) = 6x - 1 Find f · g.

Use the two given functions to write y as a function of x.

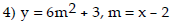
Find functions f and g so that

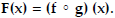
Find a formula for the inverse of the function described
below.

6) A size-34 dress in Country C is size 5 in
Country D. A function that converts dress
sizes in Country C to those in Country D is

Find the inverse of the function.

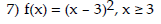
Find the difference quotient, for the
function and simplify it.

8) f(x) = 2x - 13

Graph the function.

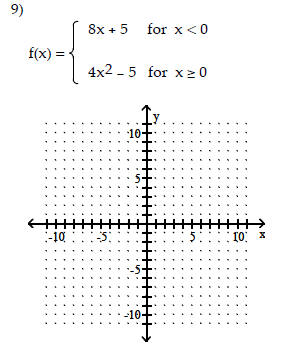
Find the domain and range.

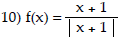
Use transformations to graph the function and state the
domain and range.

Find the specified domain.

12) For f(x) = x^2 - 64 and g(x) = 2x + 3, what is the
domain of f - g?

13) For 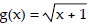and 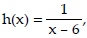what is the domain of

Find the inverse of the function.

Graph the function as a solid curve and its inverse as a
dashed curve.

Find the inverse of the function.

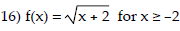
Find the maximum or minimum point of the function and
state whether it is a maximum or minimum.

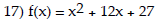
Solve the quadratic inequality by graphing an
appropriate quadratic function.

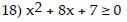
Identify the vertex of the parabola.

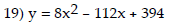
Determine whether the given number is a zero of the
polynomial function.

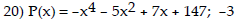
Use the rational zero theorem to find all possible rational
zeros for the polynomial function.

Use synthetic division to find the function value.

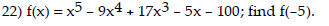
State the degree of the polynomial equation.

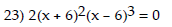
Find a polynomial equation with real coefficients that has
the given roots.

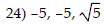
Find all real and imaginary solutions to the equation.

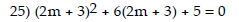
Find all real solutions to the equation.

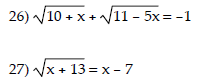
Discuss the symmetry of the graph of the polynomial
function.

28) f(x) = 7x

Use the leading coefficient test to determine whether y→
∞ or y→-∞ as x→ -∞.

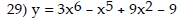
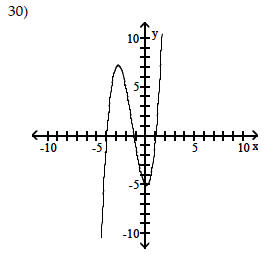
Discuss the symmetry of the graph of the polynomial
function.

Determine the domain and the equations of the
asymptotes for the graph of the rational function.

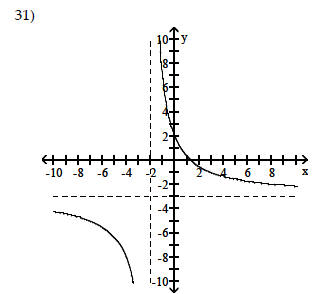
Sketch the graph of the function.